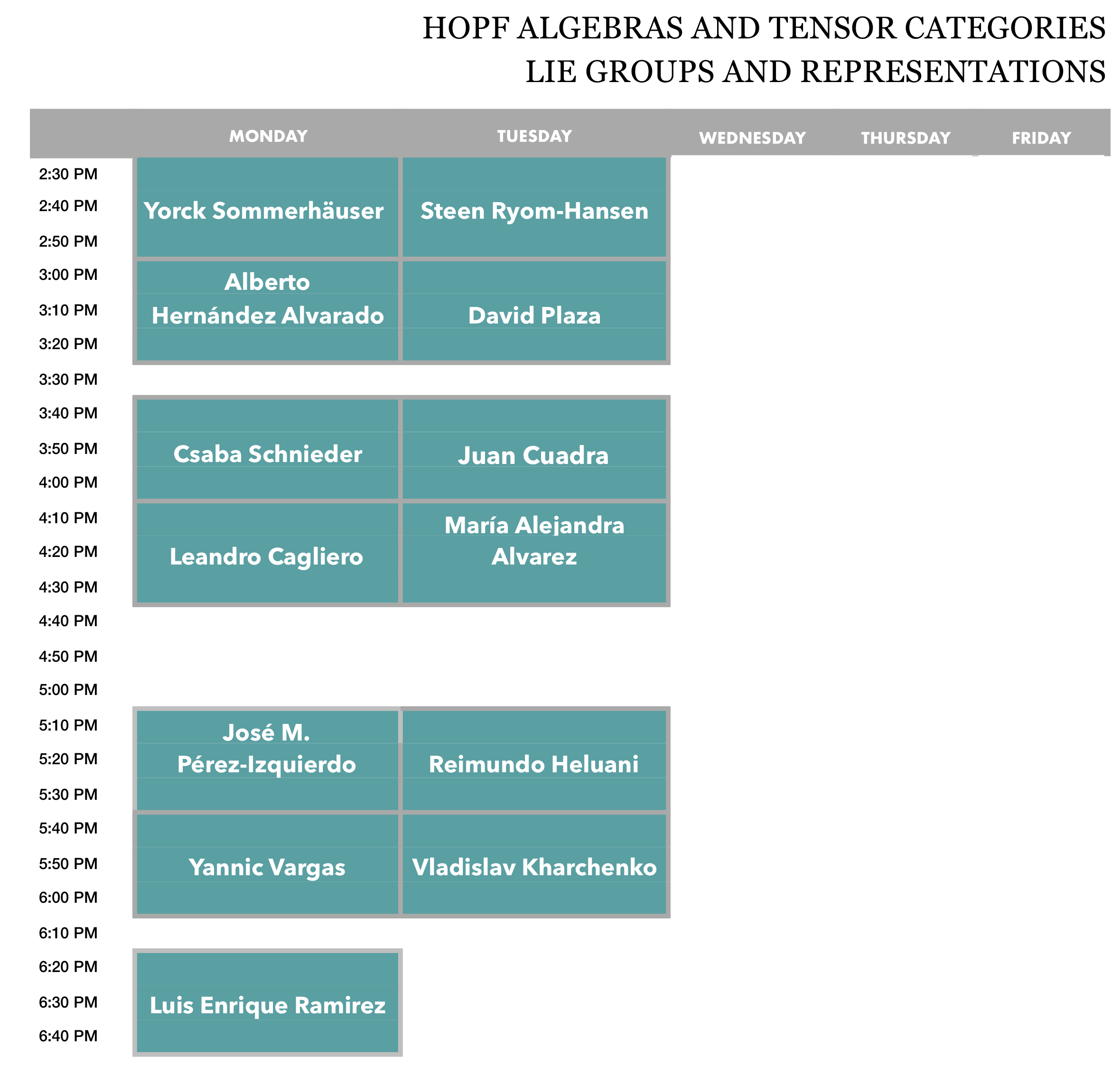
Joint program of the session on Hopf Algebras and Tensor Categories, and the session on Lie Groups and Representations:
Monday, August 5
14.30-15.00
Yorck Sommerhäuser (Memorial University of Newfoundland, Canada)
Examples of Cores in Yetter-Drinfel'd Hopf Algebras
For a finite-dimensional cosemisimple cocommutative Yetter-Drinfel'd Hopf algebra over a finite abelian group, one can define the core of a group-like element, at least when the base field is algebraically closed of characteristic zero. The core controls the action of the finite abelian group on the group-like element from inside the Yetter-Drinfel'd Hopf algebra.
In the case where the finite abelian group has prime order, the core is always completely trivial in the sense that both the action and the coaction of the group on the core are trivial. In this talk, we describe examples of Yetter-Drinfel'd Hopf algebras whose cores are not completely trivial. They have dimension 8, and their biproducts are semisimple Hopf algebras of dimension 32. The talk is based on joint work with Yevgenia Kashina.
15.00-15.30
Alberto Hernández Alvarado (CIMPA, Universidad de Costa Rica)
Minimum odd depth and the Drinfeld Double
In this paper we explore the concept of the minimum odd depth of a ring extension when the overall algebra factorises as a product of two subalgebras, in particular the case of finite dimensional Hopf algebras in their Drinfel'd double, $H\hookrightarrow D(H)$. We analyse the relationship between module depth and subring depth for this case and use it to get a result via Double Crossed Products of finite dimensional Hopf algebras. Finally we get a formula for the minimum odd depth of an extension $R\hookrightarrow H$ via the generalised smash product $Q^{*op} \#_{\psi}H$ where $Q$ is the $H$-module coalgebra associated with the extension.
15.40-16.10
Csaba Schnieder (Universidade Federal de Minas Gerais, Brasil)
The isomorphism problem for universal enveloping algebras of Lie algebras
Let $U(L)$ denote the universal enveloping algebra of a Lie algebra $L$. George Bergman in his influential article of 1978 remarked that it was an open question whether an isomorphism between two universal enveloping algebras $U(L)$ and $U(K)$ implied the isomorphism between the Lie algebras $L$ and $K$. Since the publication of Bergman’s paper we have learned that the answer to this problem is no. On the other hand, as far as we know, it is a rather rare phenomenon among small-dimensional Lie algebras that non-isomorphic algebras have isomorphic enveloping algebras. This is what makes this problem so interesting.
In this talk, I will focus on small-dimensional solvable Lie algebras. The main result I will present states that if $L$ and $K$ are solvable Lie algebras of dimension at most four over a field of characteristic zero such that $U(L)$ is isomorphic to $U(K)$, then $L$ is isomorphic to $K$. The talk is based on joint work with José Rodriguez and Hamid Usefi.
16.10-16.40
Leandro Cagliero (Universidad Nacional de Córdoba, Argentina)
Nilpotency degree of Lie subalgebras generated by two upper triangular matrices and uniserial representations
In this talk we will recall the basic facts about finite dimensional uniserial representations of Lie algebras and we will give some results about classification. In particular we will present a complete classification of all f.d. uniserial representations of the solvable Lie algebra $g = \langle x\rangle \ltimes N_k(V)$, where $N_k(V)$ denotes the free $k$-step nilpotent Lie algebra associated to a f.d. vector space $V$ and $x$ acts on V via an invertible Jordan block. A crucial step towards this classification result is obtaining the nilpotency degree of the Lie subalgebra generated by two nilpontent matrices in Jordan form $N_0$ and $N_1$, with $N_0$ of maximal degree.
17.10-17.40
José M. Pérez-Izquierdo (Universidad de La Rioja, España)
Nonassociative Solomon's descent algebra
In this talk we extend the connection between Solomon's descent algebra for the symmetric groups, the Hopf algebra of noncommutative symmetric functions and its descent algebra in the sense of F. Patras to a nonassociative setting by means of universal enveloping algebras of relatively free Sabinin algebras. We also present a nonassociative lifting of the Malvenuto-Reutenauer Hopf algebra of permutations and of the dual of the Hopf algebra of noncommutative quasi-symmetric functions. This lifting is a free nonassociative algebra as well as a cofree graded coassociative coalgebra which also admits an associative inner product that satisfies a Mackey type formula when restricted to its nonassociative Solomon's descent algebra.
17.40-18.10
Yannic Vargas (Instituto Venezolano de Investigaciones Científicas)
Hadamard product of free monoids and universals Hopf monoid
The Hadamard product $*$ is a basic operation on species which mirrors the familiar Hadamard product of power series. Aguiar and Mahajan introduced a new operation on species $\star$, based on set compositions, which intertwines with the Hadamard product via the free monoid functor. In particular, this provides an explicit basis for the Hadamard product of two free monoids in terms of bases of the factors. Using the product $\star$, we define the notion of $\star$-character of a Hopf monoid. In this work we show that the category of such elements has a terminal object, which maps via the Fock functor to a Hopf algebra based on permutations, related to the notion of permutation patterns.
18.20-18.50
Luis Enrique Ramirez (Universidade Federal do ABC, Brasil)
On the construction of admissible $\mathfrak{gl}(n)$-modules
The idea of the talk is to describe an effective method of constructing explicitly Gelfand -Tsetlin modules for $\mathfrak{gl}_n$ (relation modules). Such modules arise in [FRZ19] by allowing more general relations between basis elements in the classical construction of basis for simple finite dimensional $\mathfrak{gl}_n$-modules. We obtain a large family of simple modules that have a basis consisting of Gelfand-Tsetlin tableaux and the action of the Lie algebra is given by the Gelfand-Tsetlin formulas. This talk is based in [FRZ19].
[FRZ19] V. Futorny, L.E. Ramirez, J. Zhang, Combinatorial construction of Gelfand-Tsetlin modules for $\mathfrak{gl}_n$, Advances in Mathematics, Vol 343, 681-711 (2019).
Tuesday, August 6
14.30-15.00
Steen Ryom-Hansen (Universidad de Talca, Chile)
Graded representation theory of the higher level Temperley-Lieb algebra
In the talk we present joint work with Diego Lobos. We start out by recalling the origin and philosophy of $ \mathbb Z$-graded representation theory, where we put special emphasis on the Brundan-Kleshchev and Rouquier isomorphism Theorem between the cyclotomic KLR-algebra and the cyclotomic Hecke algebra. We explain how one can use this Theorem to obtain a non-trivial $ \mathbb Z$-grading on a certain higher level Temperley-Lieb algebra, ``the generalized blob algebra $b_n$'' that was introduced by Martin and Woodcock.
15.00-15.30
David Plaza (Universidad de Talca, Chile)
Type $\tilde{C}$ Temperley-Lieb algebra quotients and Catalan combinatorics
In this talk we explain some algebraic and combinatorial features of two algebras that arise as quotients of Temperley-Lieb algebras of type $\tilde{C}$, namely, the two-boundary Temperley-Lieb algebra and the symplectic blob algebra. We provide a monomial basis for both algebras. The elements of these bases are parameterized by certain subsets of fully commutative elements. We enumerate these elements according to their affine length.
15.40-16.10
Juan Cuadra (University of Almería, Spain)
Orders of Nikshych's Hopf algebra
Let $p$ be an odd prime number and $K$ a number field containing a primitive $p$-th root of unity. Consider Nikshych's non group-theoretical Hopf algebra $H_p$ as defined over $K$ [2]. In this talk, we will present the following result and outline its proof:
The Hopf algebra $H_p$ admits a Hopf order over the ring of integers $\mathcal{O}_K$ if and only if there is an ideal $I$ of $\mathcal{O}_K$ such that $I^{2(p-1)} = (p)$. As a consequence, if a Hopf order exists, $K$ can not be a cyclotomic field.
This provides an example of semisimple Hopf algebra over a number field not admitting a Hopf order over any cyclotomic ring of integers.
The above result appears in [1]. It is part of a more extensive investigation about Hopf orders in non-commutative/non-cocommutative semisimple Hopf algebras carried out in collaboration with Ehud Meir (University of Aberdeen, UK).
References:
[1] J. Cuadra and E. Meir, Orders of Nikshych's Hopf algebra. J. Noncommut. Geom. 11 (2017), 919-955.
[2] D. Nikshych, Non group-theoretical semisimple Hopf algebras from group actions on fusion categories. Selecta Math. (N.S.) 14 (2008), 145-161.
16.10-16.40
María Alejandra Alvarez (Universidad de Antofagasta, Chile)
Sobre las degeneraciones de superálgebras de Lie
En este trabajo, damos condiciones necesarias para la existencia de degeneraciones entre dos superálgebras de Lie complejas de dimensión $(m|n)$. Como aplicación estudiamos la variedad de superálgebras de Lie complejas de dimensión $(2|2)$, obtuvimos su clasificación algebraica y que esta variedad es la unión de siete componentes irreducibles, una de los cuales es la clausura Zariski de la órbita de una superálgebra de Lie nilpotente.
Alvarez M.A., Hernández I.: On degenerations of Lie superalgebras. Linear Multilinear Algebra. (2018). https://doi.org/10.1080/03081087.2018.1498060
17.10-17.40
Reimundo Heluani (IMPA, Brasil)
Vertex algebras and arc spaces
To each vertex algebra $V$ one can attach a graded commutative algebra with a derivation $A$. Its degree zero piece $A_0$ is a Poisson algebra. We investigate the relation between the spectrum of $A$ and the arc space of $A_0$. For several years it was not known whether these two spaces were always equal, we give examples and counterexamples to this claim. For admissible level affine Kac-Moody algebras and their quantum Hamiltonian reduction we conjecture that these spaces are equal if and only if the level is boundary admissible. We give some weak evidence for this conjecture.
17.40-18.10
Vladislav Kharchenko (UNAM, México)
Quantizations as quadratic algebras
We prove that the multiparameter quantizations of type $A_n$ and $B_n$ are quadratic-linear Koszul algebras in $q$-Weyl generators.