To view the recording of a talk, click on its title.
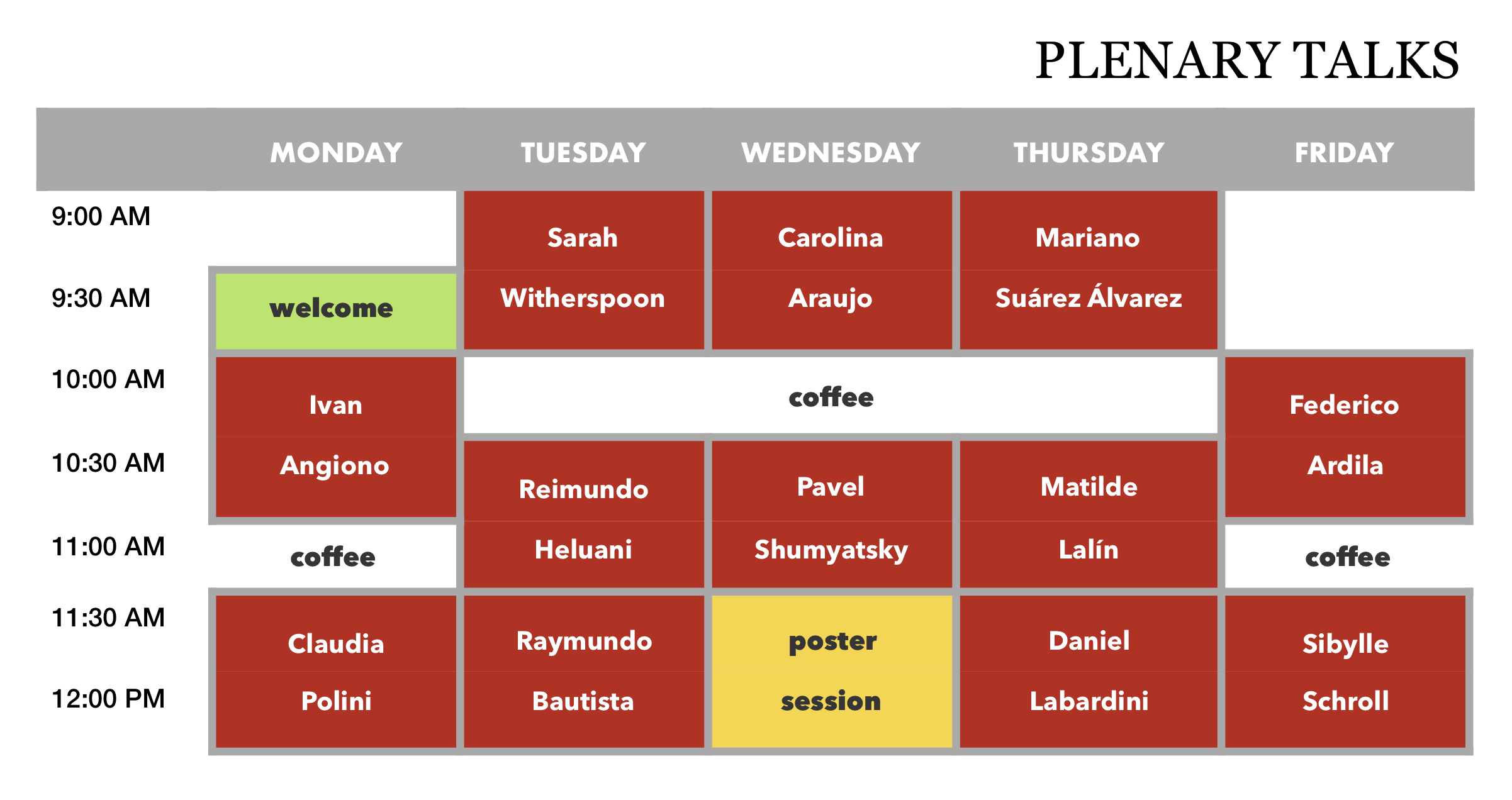
Plenary talks (at the Colegio Nacional):
Monday, August 5
10.00-11.00
Iván Angiono (Universidad Nacional de Cordoba)
Poisson orders on large quantum groups
In the nineties De Concini, Kac and Procesi introduced and studied a quantized enveloping algebra $U_{q}(\mathfrak{g})$ (where $\mathfrak{g}$ is a finite-dimensional simple Lie algebra) at a root of unity $q$. The representation theory of $U_{q}(\mathfrak{g})$ was described from a geometric approach based on the fact that $U_{q}(\mathfrak{g})$ is module-finite over a central Hopf subalgebra $Z_{q}(\mathfrak{g})$. A key ingredient of this approach is the existence of a Poisson structure on $Z_{q}(\mathfrak{g})$ so that the algebraic group $M$ corresponding to this algebra is a Poisson algebraic group, whose Lie bialgebra is dual to the standard Lie bialgebra structure on $\mathfrak{g}$. The approach consists in packing the irreducible finite-dimensional representations of $U_{q}(\mathfrak{g})$ along the symplectic leaves of $M$.
Based on a joint work with Nicolás Andruskiewitsch and Milen Yakimov, we will describe the representation theory of a larger class of Hopf algebras by means of Poisson orders. The keystone of the definition of these Hopf algebras, called large quantum groups, is the notion of distinguished pre-Nichols algebra, a covering of a finite-dimensional Nichols algebra. The Hopf algebras dealt with are module-finite over a central Hopf subalgebra. We will see that the maximal spectrum of this central Hopf subalgebra is a solvable algebraic Poisson group $M$, every irreducible representation of a large quantum group is finite-dimensional and we will describe the role played by the symplectic leaves of M.
11.30-12.30
Claudia Polini (University of Notre Dame)
Abstract: In this talk we relate the degrees of vector fields in projective n-space to properties of curves or even varieties that they leave invariant. We will survey some of the numerous previous results and report on recent joint work with Marc Chardin, Hamid Hassanzadeh, Aron Simis, and Bernd Ulrich, where the question is approached from a more algebraic point of view. We provide lower bounds for the degrees of vector fields in terms of local and global data of the curves they leave invariant. Higher dimensional varieties are considered as well, and the sharpness of the bounds will be discussed.
Tuesday, August 6
9.00-10.00
Sarah Witherspoon (Texas A&M University)
Varieties for representations and tensor categories
Algebraic geometry is used in representation theory to uncover information through the assignment of support varieties to modules. This theory began with finite group representations, and has been generalized in many directions. In this talk we will introduce the general theory in the contexts of finite dimensional Hopf algebras and finite tensor categories. These include representations of finite group algebras, restricted Lie algebras, and small quantum groups. We will discuss applications and recent developments.
10.30-11.30
Reimundo Heluani (IMPA)
The first chiral homology group
For a vertex algebra $V$, Beilinson and Drinfeld introduced a complex of vector bundles with connections on the moduli space of smooth curves. In the case of elliptic curves, the flat sections of the homology in degree 0 agree with the space of conformal blocks. Zhu proved that under certain conditions, the characters of irreducible representations form a basis of this space. We generalize his techniques for higher homology groups. In particular we associate flat sections in degree one to certain extensions of modules. This is joint work with Jethro van Ekeren.
11.30-12.30
Raymundo Bautista (UNAM, México)
Bocs is an acronym for (b)-imodule (o)ver a (c)ategory with (c)oalgebra (s)tructure. In this talk a bocs is a quadruple $\mathcal{B}=(S, W, \mu , \epsilon )$, where $S$ is an algebra over some field $k$, $W$ is a $S$-$S$-bimodule $\mu :W\otimes _{S}W\rightarrow W$ is a co-associative co-multiplication and $\epsilon :W\rightarrow S$ is a co-unit. Bocses were introduced in 1980 by A.V. Roiter.
During the talk we recall the definition and properties of the category of representations of the bocs $\mathcal{B}$, and how this category was used by Yu.A. Drozd for proving the Wild-Tame theorem for finite-dimensional $k$-algebras. Then we consider the case in which $W$ is obtained by the bar construction of some $A$-infinite algebra $A$. In this case we will see the relation between the category of representations of $\mathcal{B}$ and the $A$-infinite modules over $A$. Finally we explain how this construction is used for proving the wild tame dichotomy for the category of the stratified modules over a quasi-hereditary algebra.
Wednesday, August 7
9.00-10.00
Carolina Araujo (IMPA)
Tsen's Theorem and higher Fano manifolds
In 1936, Tsen proved that a 1-dimensional family of hypersurfaces of degree $d$ in complex projective $n$-space always admits a section provided that $d\leq n$. This simple statement has been generalized in many ways, and still inspires developments in algebraic geometry. In this talk, I will survey the history of Tsen’s Theorem, mostly from the geometric point of view, and describe current research toward new interpretations and generalizations. In particular, motivated by a conjectural generalization of Tsen’s Theorem, I will introduce higher Fano manifolds and discuss their intrinsic geometry.
10.30-11.30
Pavel Shumyatsky (Universidade de Brasília)
Centralizers in profinite groups
I will survey some recent results on centralizers in profinite groups. In particular, I will describe the theorem that if $G$ is a profinite group in which all centralizers are abelian, then $G$ is either virtually abelian or virtually pro-$p$. This is a joint result with Pavel Zalesski and Theo Zapata.
Thursday, August 8
9.00-10.00
Mariano Suárez Álvarez (Universidad de Buenos Aires)
Hochschild cohomology and its algebraic structure
10.30-11.30
Matilde Lalín (Université de Montréal)
L-functions and Mahler measure: number theory and beyond
In this talk we will begin by discussing zeta and L-functions and explaining why they are important. We will then introduce the Mahler measure, which is a function on the roots of polynomials but can also be extended to multivariable polynomials by doing a complex integral. These two seemingly unrelated topics are surprisingly connected, since the Mahler measure of multivariable polynomials often yields special values of L-functions. We will discuss some aspects of these intriguing relations as well as other unexpected relations arising from Mahler measure.
11.30-12.30
Daniel Labardini (UNAM)
Cluster algebras, hyperbolic geometry, and generic bases
Cluster algebras were invented by Sergey Fomin and Andrei Zelevinsky almost 20 years ago. Ever since, a lot of the related research has meandered between the discovery of connections with several different areas of Mathematics (e.g. Hyperbolic Geometry and Teichmüller Theory), and the search and understanding of various types of bases for cluster algebras.
I will start this talk by giving a brief overview of what a cluster algebra is (a ring whose generators are produced recursively by applying a very simple combinatorial operation, called mutation, on oriented graphs). Then I will present an identity discovered to hold in the hyperbolic plane by Robert Penner, and describe how this identity allows cluster algebras to appear as coordinate rings of Teichmüller spaces of punctured surfaces, as discovered by Sergey Fomin, Michael Shapiro and Dylan Thurston, and Vladimir Fock and Alexander Goncharov. Then I will sketch an elementary proof, obtained by Christof Geiss, Jan Schröer and myself, that certain proposed sets are bases, called generic bases, for the cluster algebras that appear in the context of punctured surfaces with non-empty boundary.
Friday, August 9
10.00-11.00
Federico Ardila (San Francisco State University)
El álgebra y la geometría de las matroides
La teoría de matroides es una teoría combinatoria de la independencia que viene del álgebra lineal y la teoría de grafos, y tiene conexiones importantes con muchos otros campos de la matemática. Con el tiempo, las raíces geométricas de esta área se han profundizado y fortalecido enormemente, dando muchos frutos.
El enfoque algebraico y geométrico a las matroides ha llevado al desarrollo de matemática fascinante, y ha brindado las herramientas necesarias para la solución a varios problemas abiertos clásicos. Esta charla dará un resumen de algunos logros recientes.
La charla no asumirá conocimiento (¡o interés!) previo sobre la teoría de matroides. Presentará el trabajo de muchas personas, incluyendo mis trabajos con Carly Klivans, Graham Denham, y June Huh.
11.30-12.30
Sibylle Schroll (University of Leicester)
Geometric surface models in representation theory
Over the past ten years, geometric surface models have played an increasingly important role in the representation theory of finite dimensional algebras. In this talk we will give a brief overview of the different models and then focus on geometric models for a well-known class of algebras, the so-called gentle algebras. While gentle algebras originate in the representation theory of algebras they appear in many different areas of mathematics, such as in $N=2$ gauge model theories, in the theory of cluster algebras and most recently in the context of homological mirror symmetry of 2-dimensional manifolds. We will introduce the geometric models for gentle algebras from a representation theoretic point of view and show how they relate to the surface models in the homological mirror symmetry program.